Is it possible to calculate your power without a power meter

Gav888

Hi,

Is it possible to calculate your power output without using a power meter, even if its a rough estimate?

I would love to know what im able to produce but I dont have a power meter... but I found the below on a cycling forum which gives approx figures, but ive no idea how accurate they are or how they were worked out as they are based on speed!?!?!

If they are talking about your average speed, then im a 145 watt pussy if they are talking a sprint then im a 650 watt monster!!

14 mph = 130 watts
15 mph = 145 watts
16 mph = 160 watts
17 mph = 185 watts
18 mph = 210 watts
19 mph = 235 watts
20 mph = 265 watts
21 mph = 295 watts
28 mph = 600 watts
30 mph = 700 watts

skavanagh.bikeradar

I asked something similar a while ago:

http://www.bikeradar.com/forum/viewtopi ... highlight=

zammmmo

Regarding those mph-watts figures - up to 20mph they seem very roughly accurate for someone on a standard road bike in my opinion. After that they look very unaccurate I mean 600 watts for 28mph? - probably more like 400-450.

jgsi

I quite like the toy calculators on this
http://bikecalculator.com/

Useful as a guide

Gav888

Thanks for the info guys... i remembered reading a thread about power and did a quick search and couldnt find anything, so thanks for the links

avoidingmyphd

there is a plugin for sporttracks which claims to do this relatively accurately.
it needs to know your bike and tyre data etc and combines them with weather info to analyse your rides.

never used it though...

Anonymous

Meh, it's inaccurate postulating without a power meter.

Unless you have a powermeter don't worry about power!

volvicspar

I put my stats into that calculator above up my local "tough" hill and it said I am outputting 800watts :P I don't think that is right!

BeaconRuth

Gav888 wrote:

Is it possible to calculate your power output without using a power meter, even if its a rough estimate?

No. I wouldn't even bother trying. But if you can't afford to buy a powermeter you could always hire one for a while. It really isn't very expensive in the grand scheme of things. This is the place to go: http://www.cyclepowermeters.com/

Ruth

Alex_Simmons/RST

The only time one can estimate power output is on steep hillclimbs, where many of the uncontrollable variables are reduced somewhat and a large proportion of the power is going to overcome gravity. Even then, it is only a guesstimate. There are many, many variables, meaning power measurement is really the only way to know.

JohnWilky

Although there are some who have developed some models for estimating;

http://analyticcycling.com/ForcesPower_Page.html

John.

Alex_Simmons/RST

JohnWilky wrote:

Although there are some who have developed some models for estimating;

http://analyticcycling.com/ForcesPower_Page.html

John.

Yes, but you then need to know all of the variables in question, which unless you've been to a wind tunnel, or have a power meter in the first place, you'll never really know and any such numbers will be, at best, rough guesstimates.

Also AC doesn't provide for a wind vector.

Putting wind aside, as an example, I rider I coach set a new masters world record for the hour a few weeks ago. 48.317 km. His average power was 302 watts.

At 300W, I would travel maybe 42 km/h on the same velodrome.

Or put it another way:

At 48.3km/h he does 302W
At the same speed I need to do 440W.

On a dead flat road, no wind, on my road bike, to do 48.3km/h, I'd need to put out well in excess of 500 watts.

But this is because:
a. I know what the variables are for myself and my riders
b. I use power meters

Hence, unless you know all of the variables, there's not much point speculating.

BTW - I've developed same model (except I solved the equations in excel so don't need to use Tom's excellent website tools).

James_London

You guys are a little negative on the OP's request...

If you want to know 'what you are able to produce' do the longest climb you can find at your maximum sustainable pace and use Friel's simple formula to calculate your average power:

http://www.trainingbible.com/joesblog/2 ... rmula.html

I've just looked at the prediction versus my SRM data for a few long climbs and it's within 2% which whilst it isn't perfect, gives you a reasonable idea. Then have a look where that puts you in terms of Watts/Kg here:

http://home.trainingpeaks.com/articles/ ... iling.aspx

If you can get a 20 minute climb you can get an idea of your FTP (95% of your 20 minute average as a guide) which is really what you want to know but you can look at 5 minute in the table.

Alex - As an aside, I was surprised by the average power for that hour record. Whilst the guy's tiny, 4.0 W/Kg isn't that special. Is the record not that hotly contested?

Alex_Simmons/RST

James_London wrote:

You guys are a little negative on the OP's request...

Hey, I already said a steep hillclimb was the only place one can make such as estimate.

James_London wrote:

Alex - As an aside, I was surprised by the average power for that hour record. Whilst the guy's tiny, 4.0 W/Kg isn't that special. Is the record not that hotly contested?

His record was set at 5.0 W/kg for the hour.

But more importantly, it was set at a Power to CdA ratio of ~1630W/m^2 and it is this latter number that primarily dictates how relatively fast you'll be in a time trial, not power to mass.

If you think it's not hotly contested, drop on down to your local 250 metre velodrome and ride some 18.6 second laps. Now do it for an hour.

James_London

Apologies - I misread and thought the 259W NP was for the record.

Clearly it's all about CdA, it's just that pro FTP is more readily available than CdA and whilst 5W/Kg is decent, I thought there must be quite a few 60kg or lower pros who could achieve similar CdA with a superior FTP.

Whilst my W/Kg is respectable, my CdA, is far too high so I had no intention of contesting it myself!! And nor did I mean to belittle the achievement - good on him!

Bhima

Gav888 wrote:

14 mph = 130 watts
15 mph = 145 watts
16 mph = 160 watts
17 mph = 185 watts
18 mph = 210 watts
19 mph = 235 watts
20 mph = 265 watts
21 mph = 295 watts
28 mph = 600 watts
30 mph = 700 watts

18mph in a headwind requires a lot more power than 18mph in a tailwind. So you'll never get an accurate estimate for any mph figure which will work 100% of the time.

That climbing formula seems to be pretty accurate, but surely you could cut down on external forces even more by doing it on a trainer...?

The only problem I see is that, using that formula, on a trainer, the elevation gain would be zero - which would mess up the calculation.

Can you use E=mc^2 to calculate energy, therefore power?

eg. Energy = Bike+Rider Mass x Speed^2

I think you'd need to introduce time into the equation somehow to calculate power, although i'm not too sure how...

:?:

DaveyL

You're kidding, right?

chrisw12

Bhima wrote:

Gav888 wrote:

14 mph = 130 watts
15 mph = 145 watts
16 mph = 160 watts
17 mph = 185 watts
18 mph = 210 watts
19 mph = 235 watts
20 mph = 265 watts
21 mph = 295 watts
28 mph = 600 watts
30 mph = 700 watts

18mph in a headwind requires a lot more power than 18mph in a tailwind. So you'll never get an accurate estimate for any mph figure which will work 100% of the time.

That climbing formula seems to be pretty accurate, but surely you could cut down on external forces even more by doing it on a trainer...?

The only problem I see is that, using that formula, on a trainer, the elevation gain would be zero - which would mess up the calculation.

Can you use E=mc^2 to calculate energy, therefore power?

eg. Energy = Bike+Rider Mass x Speed^2

I think you'd need to introduce time into the equation somehow to calculate power, although i'm not too sure how...

:?:

'Can you use E=mc^2 to calculate energy, therefore power?'

I don't look at this forum (or post much) I'd rather train, but that quote is priceless.

Einstein must be turning in his grave.

Bhima

As far as I know, E=mc^2 will tell you how much energy is required to accellerate a given mass to a given speed...?

Correct?

If so, you could work out the amount of energy a rider needs to accelerate himself+bike to a given speed. Of course, that would be if the rider was in a vaccum, which is why the idea of a trainer came in... Then again, there is no "speed" on a trainer, unless you can measure the resistance exactly...

Can't remember exactly what it was, but swear i've seen an equation somewhere which allows you to work out power from energy + some other figures...

Perhaps i've misunderstood something somewhere... :?

I'm baffled - been a while since i've used the physics part of my brain. Can someone clear this up then, instead of mocking my quotes? :roll:

Alex_Simmons/RST

E=mc^2 is too simple a formula.

Here is the formula I use to solve power for speed or speed for power: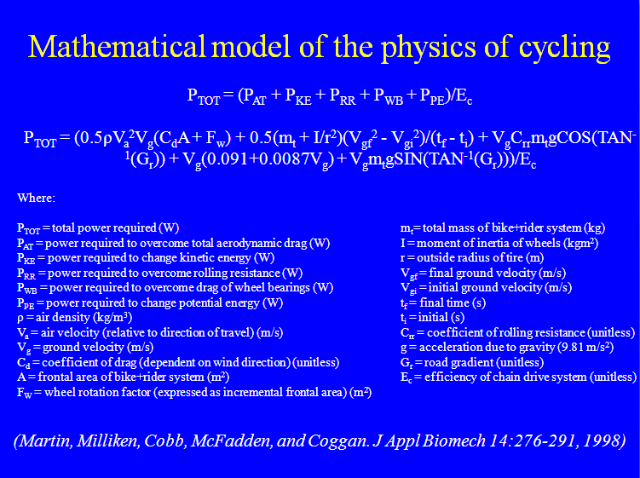



It works exceptionally well.

Alex_Simmons/RST

Bhima wrote:

As far as I know, E=mc^2 will tell you how much energy is required to accellerate a given mass to a given speed...?

Correct?

No

Bhima wrote:

Perhaps i've misunderstood something somewhere... :?

Yes

Bhima wrote:

I'm baffled - been a while since i've used the physics part of my brain.

Clearly.

Bhima wrote:

Can someone clear this up then, instead of mocking my quotes? :roll:

Refer my previous post. They are the correct formula.

chrisw12

Bhima, sorry to mock but you are so far away with e=mc^2 as to be out of this universe.

Go and do some reading, you might surprise yourself, and you'll also understand why we're laughing.

Slow1972

Priceless, good to see Bhima's back, that's ended my working week with a laugh

joeyhalloran

e=mc^2 is the contained energy within a given mass. Its the ratio of energy:mass conversion. not really sure if it would come in to cycling. Do you mean F=ma i.e. mass-force x acceleration? In which case, obviously, you have to be accelerating.

bompington

E=mc^2 tells us that if you annihilated me and my bike completely in a nuclear explosion, you could get 30000000000000000 Joules of energy, or enough to keep a kettle boiling for about 31 million years. Of course if you did this to a weightweenie on a serious carbon bike, you're looking at more like half an hour.
I think the formula you're really looking for is E = 1/2mv^2, which is the amount of kinetic energy a body of mass m has when going at a speed v. This would indeed be the amount of energy needed to accelerate to that speed in a perfect world, without forces like friction or air resistance to deal with.
That doesn't really have anything to do with the energy needed to maintain a constant speed against these forces. Alex's impressive looking formula is actually mathematically quite simple & basically calculates & adds up the resistive forces - drivetrain inefficiency, rolling resistance on the road, rolling resistance in the wheel bearings, and of course the biggest: air resistance.
Now I don't know the precise proportion of the total resistance that air resistance gives (I'm sure someone round here does, so let me know), but I'd be prepared to bet that at any serious cycling speed (say over 20mph) it's the vast majority.
The power needed to overcome air resistance is proportional to v^3 - or to put it more simply, to go 2X faster needs 8X more power (a Bugatti Veyron can go twice as fast as my Skoda, with an engine that is indeed about 8X more powerful).
This also explains why it takes significantly more power to increase your speed by quite small amounts - 21mph is 5% faster than 20mph but the power needed to overcome air resistance goes up by nearly 16%, and 25mph requires roughly double the power that 20mph does - which tallies fairly well with the table quoted by the OP.

Mettan

bompington wrote:

This also explains why it takes significantly more power to increase your speed by quite small amounts - 21mph is 5% faster than 20mph but the power needed to overcome air resistance goes up by nearly 16%, and 25mph requires roughly double the power that 20mph does -

Interesting - heard about the mid/late 20's to 20 diff - amazing seeing the 20/21 difference though.

Alex_Simmons/RST

bompington wrote:

Alex's impressive looking formula is actually mathematically quite simple & basically calculates & adds up the resistive forces - drivetrain inefficiency, rolling resistance on the road, rolling resistance in the wheel bearings, and of course the biggest: air resistance.

It isn't my formula. it's from the following 1998 reference:
Validation of a Mathematical Model for Road Cycling Power
by James Martin, Douglas Milliken, John Cobb, Kevin McFadden and Andrew Coggan

You are right though, it looks worse than it actually is.

The trickiest bit is when you try to solve for speed from power (and other assumptions), as you need to (for steady state efforts) solve a cubic equation, or if you are talking about non steady state efforts (i.e. including accelerations), use a forward integral method.

bompington

True enough Alex, ain't maths great?
You sound like the sort of person who could answer my query about the proportion of total resistance caused by air resistance at different speeds; and maybe an interesting consequence - has anyone actually measured, in any kind of objective way, the differences in things like drivetrain efficiency & wheel bearing resistance between different bikes / components - especially when plotted against cost...

Alex_Simmons/RST

bompington wrote:

True enough Alex, ain't maths great?
You sound like the sort of person who could answer my query about the proportion of total resistance caused by air resistance at different speeds; and maybe an interesting consequence - has anyone actually measured, in any kind of objective way, the differences in things like drivetrain efficiency & wheel bearing resistance between different bikes / components - especially when plotted against cost...

I could but I didn't build my models that way to specifically show the relative contributions to resistance (since I am more interested in solving speed from power).

Others have done that though, I can't specifically recall right now where one of those examples might be. it's pretty easy though - it's just the listed formula for each element.

As for testing drivetrain efficiency, well that's a little tricky but one can inspect the power data from calibrated SRM and Powertap power meters installed on the same bike, and note the difference in power. repeat on another machine with same meters and note the variances in power differential. Bearings are only tiny part of it - it's mostly the drivetrain.

efficiency can also vary depending on the chainring and cog selected, as there is a relationship with chain tension/chain speed, although I have seen conflicting data as to which way that relationship operates.