Estimating Power

bilbo.baggins

This has maybe been answered somewhere, so apologies in advance, as I haven't been able to find it on the forums.

I'm looking for a 'rough and ready' power measurement without the expense and hassle of fitting a power meter. I'm thinking that if I have all the measurements below it should be possible to estimate average power output for a steady climb, and hence compare different techniques, but don't know the equations to use. (I realise this will be an estimate only as wind direction and road condition, etc, will all make a difference).

Example stats
Rider Weight: eg. 70 kilos
Bike Weight: eg. 9 kilos
Length of climb: eg. 2 km
Height gained: eg. 150m
Time taken: eg. 300 secs
Average cadence: 75 rpm
Gear: 66.3 inches (sorry for the imperial)

Thanks

nasahapley

Easy peasy mate, power is just energy expended over time. In this case the change in potential energy is given by mgh, or mass (in kilos) x gravity (in metres per second) x change in altitude (in metres), so:

Change in potential energy =

79 x 10 (or 9.81 if your really picky) x 150 = 118500 joules,

divide by time (in seconds):

118500/300 = 395 watts.

So given the figures below your power would average at least 395 watts for the climb; you could add a bit on (dunno how much - I'd guess 5-10%) for mechanical drag, so maybe around 420 watts. Not bad!

ColinJ

nasahapley wrote:

Easy peasy mate, power is just energy expended over time.

I know what you're saying, but strictly it is the other way round !

Power is the rate of expenditure of energy i.e. energy is power expended for a period of time.

Ooops, I've just reread your post... that's what you said :oops:

(PS since you didn't turn up for my forum ride on the 17th, I assume that your mate's birthday bash went rather well!)

bilbo.baggins

nasahapley wrote:

Easy peasy mate, power is just energy expended over time. In this case the change in potential energy is given by mgh, or mass (in kilos) x gravity (in metres per second) x change in altitude (in metres), so:
....
So given the figures below your power would average at least 395 watts for the climb; you could add a bit on (dunno how much - I'd guess 5-10%) for mechanical drag, so maybe around 420 watts. Not bad!

Many thanks. Of course the figures were only examples. I'd love to be able to generate what averages out to be 6W/Kg but using my own figures I was putting out a more 'relaxed' 250W (+ mechanical drag).

Alex_Simmons/RST

bilbo.baggins wrote:

but don't know the equations to use.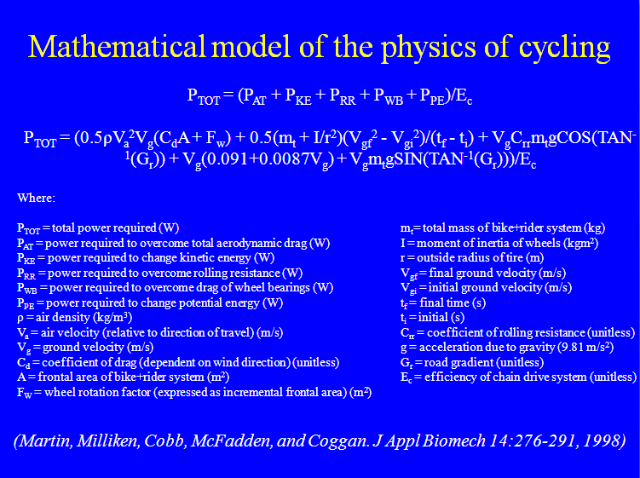

This is about as good as it gets:



Solving for power with the above isn't as hard as it first looks. The hard bit is knowing the variables. For climbing it is most a change in potential energy with some aero drag and rolling resistance. You can assume negligible change in kinetic energy (acceleration).

The tricky bit is solving the equations for speed given power (you'll need to solve some cubic equations), which I've done for a bit of fun.

Or you could just use http://www.analyticcycling.com/

milton50

Alex_Simmons/RST wrote:

bilbo.baggins wrote:

but don't know the equations to use.

This is about as good as it gets:



Solving for power with the above isn't as hard as it first looks. The hard bit is knowing the variables. For climbing it is most a change in potential energy with some aero drag and rolling resistance. You can assume negligible change in kinetic energy (acceleration).

The tricky bit is solving the equations for speed given power (you'll need to solve some cubic equations), which I've done for a bit of fun.

Or you could just use http://www.analyticcycling.com/

I thought you'd have to take into acccount the gradient of the road.

meenaghman

This site can perform some of the calculations you want also.

http://www.kreuzotter.de/english/espeed.htm

you may have to clear speed/power boxes before re-calculating.

portuguese mike

Milton50 wrote:

Alex_Simmons/RST wrote:

bilbo.baggins wrote:

but don't know the equations to use.

This is about as good as it gets:



Solving for power with the above isn't as hard as it first looks. The hard bit is knowing the variables. For climbing it is most a change in potential energy with some aero drag and rolling resistance. You can assume negligible change in kinetic energy (acceleration).

The tricky bit is solving the equations for speed given power (you'll need to solve some cubic equations), which I've done for a bit of fun.

Or you could just use http://www.analyticcycling.com/

I thought you'd have to take into acccount the gradient of the road.

erm it does - its the penultimate variable on the list

milton50

Yeh, it's hard to get tone across when writing. It was in response to nasahapley's original equation which didn't involve gradient.

portuguese mike

Milton50 wrote:

Yeh, it's hard to get tone across when writing. It was in response to nasahapley's original equation which didn't involve gradient.

Sorry, i realise that now reading it back

Alex_Simmons/RST

portuguese mike wrote:

Milton50 wrote:

Yeh, it's hard to get tone across when writing. It was in response to nasahapley's original equation which didn't involve gradient.

Sorry, i realise that now reading it back

Quoting the right post helps.

milton50

Yep.

nasahapley

Right now that little bit of confusion's sorted out, I'll try to explain how the formula I posted does in fact take gradient into account (though this may be difficult without being able to use mathematical notation!)

The formula I used to calculate power - (mass x gravity x height)/time, plus a little bit for mechanical drag and air resistance, is just a simplified version of Alex's formula. mgh/t in my formula equates to the 'power required to change potential energy' bit in Alex's (i.e. the last bit inside the bracket).

In Alex's formula to find the power requied to change potential energy you multiply mass x gravity x speed x sin(tan-1 gradient). Now we know speed is distance travelled per unit time (i.e. per second), so speed x sin(tan-1 gradient) is just another way of saying height gained per unit time, or h/t, so mass x gravity x speed x sin(tan-1 gradient) can be re-written as mgh/t. Gradient is only used in Alex's formula as a means of working out the height gained per unit time, my formula just assumes you know the height gained already.

Where my formula differs quite obviously from Alex's is that it doesn't explicitly work out the power required to overcome mechanical drag and air resistance, it just assumes it'll be in the region of 5-10%. As Alex has already said, measuring all the relevant variables accurately is going to be very very difficult for the everyday cyclist, but because you'll not be going very fast when climbing air resistance won't be too large, and the other resistances will also be small in relation to that required to change potential energy.

Got that? Good, now write it out 100 times.

Alex_Simmons/RST

nasahapley wrote:

Got that? Good, now write it out 100 times.

No need to.

Your logic is sound, particularly on steeper grades.

Indeed it is how an estimate of Ricco's power on his TdF stage "win" was arrived at. When we all saw something like 6.5W/kg, it looked a most remarkable performance. Then the news broke of his doping infraction and it wasn't so remarkable.