Hill Climbing Speed

2»

Comments

  • GeorgeShaw
    GeorgeShaw Posts: 764
    One calculation that I filched from somewhere is:

    (total weight in kgs) x 9.8 x (elevation gain in metres) / (time in seconds) x 1.1

    9.8 is gravity (metres per second) and 1.1 is meant to represent an additional coefficient for rolling resistance, I think.
  • doyler78
    doyler78 Posts: 1,951
    GeorgeShaw wrote:
    One calculation that I filched from somewhere is:

    (total weight in kgs) x 9.8 x (elevation gain in metres) / (time in seconds) x 1.1

    9.8 is gravity (metres per second) and 1.1 is meant to represent an additional coefficient for rolling resistance, I think.

    Thanks George looks a simple enough calculation. Will give it a go and see what it comes up with :lol:
  • Alex_Simmons/RST
    Alex_Simmons/RST Posts: 4,161
    doyler78 wrote:
    Alex are you using an online calculator to work out those power estimations? If it is you couldn't give a link. :D
    Actually I have my own Excel spreadsheet as I went to the trouble to solve the equations of motion for a cyclist*.

    Power from speed is easier to calculate than speed from power, as the latter requires you to solve cubic equations, which is a little tricky but can be done with a couple of methods (Cardano and Newton).

    However it essentailly mirrors the output of what you can get by putting the assumptions into the calculators available here:
    http://www.analyticcycling.com/

    Although mine does a couple of things analytic cycling doesn't.

    Analytic Cycling is the best site for this as it doesn't make assumptions for you, like many other sites do.

    Couple of comments:

    i. For climbing steep gradients, then the power output for a given speed is not all that senstive to the Coefficient of Drag (Cd) and Effective Frontal Area (A) used.

    Cd and A are typically multiplied together to give what's known as CdA (units m^2) - a measure of how well you slice through the air. Lower the number, the slipperier you are. A good TT rider will have a CdA ~ 0.22-0.26 depending on size etc. Up on the hoods a rider might be anywhere from 0.3-0.4. On flatter terrain, this has a large impact.

    In analytic cycling these are shown as separate entry assumptions but really just set one to 1 and the other to your CdA estimate.

    ii. Also, for climbing steep gradients, again the power output given is not all that sensitive the the Coefficient of Rolling Resistance (Crr) used - a unitless measure. Lower = faster or less power required at given speed.

    Analytic Cycling does list some suggested values, however these are understated somewhat. The best Crr I've seen measured for a wooden velodrome is in the vicinity of 0.0022-0.0023 (that includes work done by the AIS, by Dr Andrew Coggan and my own testing of clients and myself).

    For normal asphalt roads and good road bike tyres, a Crr of 0.005 is typical. Tyres can vary greatly in Crr. Again, Crr makes a larger difference on flatter terrain.


    * The equations of motion I used were from the Martin et al paper and can be seen here for anyone interested: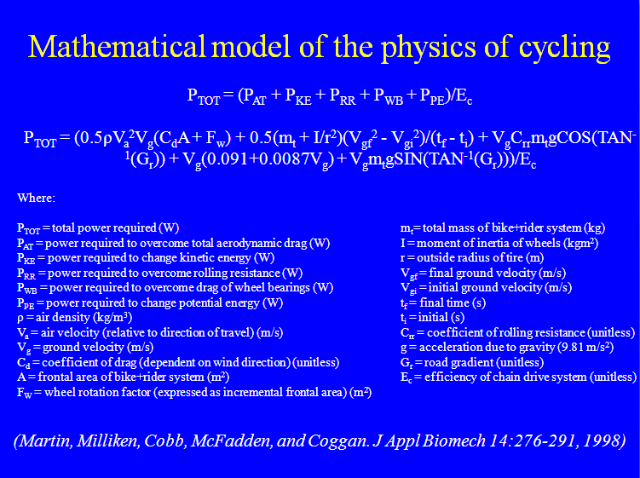
    MathModelofCycling.png

    All this has given me a bit of a thought for my next BikeRadar article.... :idea:
    RST Sport : Coaching - Training Plans - Powermeters -- My blog -- AeroCoach Australia
  • doyler78
    doyler78 Posts: 1,951
    Thanks Alex that will take a few reads to get my head round :shock:

Categories